Is the Universe a Giant Fractal?

Fractals are objects that look the same on all scales. I’m sure many of you have seen pictures or videos of fractals, but if you haven’t or if you would like a reminder, check out this visual representation posted on YouTube. As a cosmologist who has studied the large scale structure of the Universe, I find the question of whether the Universe is itself a giant fractal pretty interesting.

Before we can dive deeper into this question, some background information is required. The prevailing conclusion in cosmology is that the Universe originated in a Big Bang from which all matter and energy was set in motion. Though it was initially very close to uniform, tiny quantum perturbations made certain sections of the Universe slightly more dense than others. As gravity directed matter into these overdense regions structure slowly began to form. After billions of years this structure evolved into a massive collection of filaments and voids. The following video from the Millennium simulation displays a model of that structure on different length scales.

As the video shows, the Universe does appear somewhat similar on all scales except the smallest. That the Universe fails being a fractal at small scales should be obvious. After all, there are no galaxy-sized objects that look like glaciers, trees or chipmunks. Therefore if the Universe does possess fractal-like properties they must break down at some point. Above those scales, does the Universe look like a fractal? If so, does that fractal go on forever? If not, where does it cut off. Why? How do we know?

These are the questions I investigate in this post. Fair warning: this is about to get pretty wonky. Those valiant enough to proceed are encouraged to put on their math caps.

One way cosmologists quantify structure is through a statistic known as the two-point correlation function (2PCF). The 2PCF measures the probability of finding two galaxies separated by distance $r$ beyond what’s expected through random chance.

In three dimensions the two-point correlation function is often approximated as a power law,

(1) $\begin{equation*} \xi(r) \propto r^{-\gamma}, \end{equation*}$

where $\gamma$ is a parameter whose value depends upon the particular distribution of galaxies. In two dimensions the 2PCF $w(\theta)$ is a function of angle,

(2) $\begin{equation*} w(\theta) \propto \theta^{-(\gamma-1)}. \end{equation*}$

Note that if we add the number of Euclidean dimensions¹The Universe possesses 3 Euclidean, or topological dimensions. This is another way of saying we live a three-dimensional Universe – up/down, left/right, in/out. We distinguish between Euclidean and fractal dimensionality since the latter can take non-integer values and more accurately describes fractals’ more complicated geometric properties. to the exponent of the 2PCF we obtain the same number, $3-\gamma$ . This is known as the codimension. It turns out that if you have a random process with a power law correlation function, when you project it into lower dimensions the codimension does not change.

To put more substance behind this, let’s consider the two-point galaxy correlation function in greater depth. To compute its value at any $r$ we populate a simulated volume with uniformly distributed²In this context “uniformly distributed” means the random points must have the same distribution the observed galaxies would in the absence of large scale structure. The geometry of the survey must therefore be taken into account. No random points may be placed in locations where galaxies could not be observed. If the number of observed galaxies decreases with distance as with magnitude-limited surveys, so too must the number of randoms. random points. We count the number of pairs of points separated by each distance $r$ and use the results to populate a so-called randoms-randoms histogram. We do the same for the galaxies to generate a data-data histogram. The ratio of these histograms, which is a measure of probability above and beyond what one would expect through random chance, is the 2PCF.³For more on this see Landy, S. D., & Szalay, A. S. 1993, Astrophysical Journal, 412, 64.

As an example consider a three-dimensional Universe in which all the galaxies lie along a straight line. We limit our focus to galaxies separated by a distance $r$ by imagining a spherical shell of radius $r.$ The only data-data points would lie across the shell from each other, perhaps located at opposite poles. The number of galaxy pairs would scale as $2\lambda\, dr$ where $\lambda$ is the linear galaxy density. The random points could lie anywhere within the spherical shell, contributing to a much greater number of pairs. The number of these pairs would scale as $4\pi r^2 \rho\, dr$ where $\rho$ is the volume density of the randoms.⁴ $4\pi r^2$ is the surface area of a sphere. When multiplied by the infinitesimal thickness $dr$ it becomes the volume of a very thin spherical shell. The correlation function would then go as

(3) $\begin{equation*} \xi=\frac{2\lambda \, dr}{4\pi r^2 \rho \, dr} \propto \frac{1}{r^2}=\left( \frac{1}{r} \right)^{\gamma=2}. \end{equation*}$

By a similar argument if all the mass in the Universe was on a plane, then the number of data-data pairs would go as $2\pi r \sigma\,dr$ where $\sigma$ is the galaxy area density.⁵A plane (of galaxies in this instance) intersected with a spherical shell creates a circular ring. The circumference of that ring is $2\pi r$ . When multiplied by the ring’s thickness $dr$ we get the area of the ring. In this case the correlation function would go as

(4) $\begin{equation*} \xi=\frac{2\pi r \sigma\,dr}{4\pi r^2 \rho \, dr } \propto \frac{1}{r}= \left( \frac{1}{r} \right)^{\gamma=1}. \end{equation*}$

The codimension of the linear Universe is $3-\gamma=1$ . The codimension of the planar Universe is 2.

The reason this matters is that a random process (like the distribution of galaxies) with a power law correlation function has a lot in common with fractals.⁶Though it might seem counterintuitive, the distribution of galaxies is considered to be a random process. That is, there could be an infinite number of different Universes that each have the same 2PCF. This is analogous to many people rolling a die a large number of times. Each person will roll numbers 1 through 6 in a different order even though the probability of rolling each number is identical for all of them. In fact, simulating the positions of galaxies is sometimes referred to as rolling the dice. To see how, let’s examine the concept of dimensionality a bit more rigorously.

Imagine intersecting familiar geometric objects with a sphere and then doubling the radius of the sphere. What happens? If the object is a line, the length of the line inside the sphere will double. This means it increases by a factor of $2^{D=1}$ . If the object is a flat plane, the area of the plane inside the sphere will quadruple. This means it increases by a factor of $2^{D=2}$ . In these examples the exponent $D$ tells you the dimensionality of the object. A line is 1-dimensional. A plane is 2-dimensional.⁷I have taken the radius of the sphere to increase by a factor of 2, but note that the argument works for any factor $f$ , e.g. changing the radius of the sphere from $R$ to $fR$ scales the area of the intersecting plane by $f^{D=2}$ .

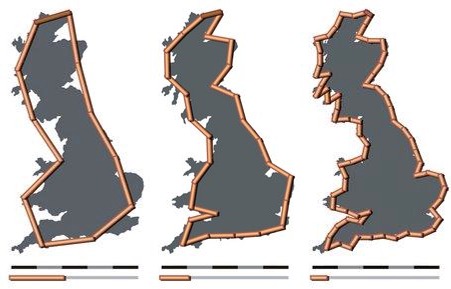
While lines and planes are relatively simple objects, the boundaries of fractals are not. In fact, the length around a fractal shape depends upon how fine a ruler one uses. For example, consider the images of the United Kingdom’s coastline below. The shoreline appears jagged on all scales and can be approximated to be a fractal. As the resolution of the ruler increases, so too does the length of the coastline. And because fractals have infinitely dense structure, the closer you look the longer the edge gets. For this reason the edges of pure fractals are often considered infinite in length.

$Britain-fractal-coastline-combined$

When you intersect a fractal with a sphere and double its radius, the spatial content of the fractal doesn’t necessarily double or quadruple – it increases by a factor $2^{F_D}$ where $F_D$ is known as the fractal dimension. And unlike in Euclidean geometry, the fractal dimension does not need to be an integer.⁸The fractal dimension is also a measure of the complexity of a fractal’s boundary. There are formal defintions of $F_D$ , but those are omitted here.

It is somewhat comforting that $F_D=1$ for a straight line and $F_D=2$ for a flat plane, i.e. for simple cases the Euclidean and fractal dimensions are identical. But if a line is somewhat curved, it will have a fractal dimension close to but greater than 1. If a line is so tangled that it almost maps out an entire area, it will have a fractal dimension close to but less than 2. A similar logic applies to surfaces. A slightly curved surface will have a fractal dimension somewhat larger than 2 while a surface so folded that it practically maps out the entire volume will have a fractal dimension somewhat smaller than 3.

The essential connection between these examples is that the codimension $3-\gamma$ and the fractal dimension $F_D$ are actually measuring the same thing. A linear Universe has a codimension of 1 and the fractal dimension of a straight line is $F_D=1$ . A planar Universe has a codimension of 2 and the fractal dimension of a plane is $F_D=2$ .

This relationship is nontrivial. Dimensionality is a measure of how the spatial extent of a geometric form scales within a volume. The codimension is a measure of how objects are distributed relative to a purely random distribution. They are fundamentally different things, yet in the context of power law 2PCF they wind up being equal.

And while these are just the edge cases, this conclusion holds equally well for $1<\gamma<2$ . In other words, if we know the two-point correlation function, we know the fractal structure of the Universe!

So if the Universe is indeed a fractal, what is its mass? The answer depends upon the radius $R$ of the sphere within which we measure it. For a sphere centered on position $x_0$ we might use an equation like this,

(5) $\begin{equation*} m_R(x_0) = \int \rho(x) W_R(x_0-x) \, d^Nx, \end{equation*}$

where $\rho(x)$ is the density at position $x$ , $W_R(x_0-x)$ is a top-hat window function⁹The top-hat window function equals 1 when $x$ is within a distance $R$ of $x_0$ and equals zero otherwise. It exists to limit the integration to the interior of the sphere. and $N$ is the dimensionality of the fractal¹⁰For conventional three-dimensional objects $N=3$ . When integrating over surfaces we use $N=2$ .. To find the average fractal mass within a radius $R$ we would average $m_R(x)$ over many positions.

Regardless of the particulars of the density function $\rho(x)$ , the mass of a fractal is proportional to length raised to the $N^{\text{th}}$ power, or $R^{F_D}$ . The mass density of a fractal therefore scales as

(6) $\begin{equation*} \rho\left(R \right)=\frac{m_R}{V} \propto \frac{R^{D_F}}{R^3}=R^{D_F-3}. \end{equation*}$

Experiments have shown that in our Universe,

(7) $\begin{equation*} \xi\left(r\right) \propto \left( \frac{1}{r}\right)^{\gamma=1.8}. \end{equation*}$

We might naively conclude from this that the fractal dimension of all space is $D_F=1.2$ . This lands close to the truth but misses an important point. When $D_F<3$ , we have $D_F-3<0$ . It therefore follows from equation 6 that as $R \rightarrow \infty, \rho \rightarrow 0$ . In other words, the mean density of a fractal with $D_F<3$ is zero.

Our Universe has a nonzero density $\rho$ , so something doesn’t quite fit. The explanation lies in the definition of the two-point correlation function. Recall that the 2PCF quantifies the probability of finding galaxies above what’s expected through random chance. If we represent the density of the Universe as the sum of a background component $\rho_{bg}$ and a perturbative component $\rho_{fr}$ above and beyond that of an expected background, we have

(8) $\begin{equation*} \rho(\mathbf{x})=\rho_{bg}+\rho_{fr}(\mathbf{x}). \end{equation*}$

The density of the Universe is not what exhibits fractal properties. Rather, it is the density $\rho_{fr}(\mathbf{x})$ atop the background that does. Because $\rho_{fr}(\mathbf{x})$ is a perturbation from the mean, it has an expected value of zero when averaged over all space,

(9) $\begin{equation*} \langle \rho_{fr} \rangle_{\mathbf{x}} = 0, \end{equation*}$

and thus satisfies the requirement that the mean density go to zero as $R \rightarrow \infty$ .

I close with the following conclusion – the Universe does behave like a fractal as long as its two-point correlation function follows a power law relationship. Where the 2PCF fails to be modeled by equation 1, the equality between the codimension and fractal dimension no longer holds and the rest of the argument breaks down.¹¹The approximation of the 2PCF as a power law works well for intermediate length scales. At small separations (e.g. the size of galaxies) the growth of structure is governed by factors far more complicated than simple gravity like supernovae, shockwaves, tidal forces, accretion disks, etc. At large separations parcels of matter are so distant that they have yet to have time to affect each other.

Featured image: “Stardust Memories” by Anua22a, used under CC BY-NC-SA 2.0 / Cropped from original
Britain fractal coastline image: originals left, middle and right made by Avsa mixed by Acadac, used under CC BY-SA 3.0

Notes[+]

Notes
↑1	The Universe possesses 3 Euclidean, or topological dimensions. This is another way of saying we live a three-dimensional Universe – up/down, left/right, in/out. We distinguish between Euclidean and fractal dimensionality since the latter can take non-integer values and more accurately describes fractals’ more complicated geometric properties.
↑2	In this context “uniformly distributed” means the random points must have the same distribution the observed galaxies would in the absence of large scale structure. The geometry of the survey must therefore be taken into account. No random points may be placed in locations where galaxies could not be observed. If the number of observed galaxies decreases with distance as with magnitude-limited surveys, so too must the number of randoms.
↑3	For more on this see Landy, S. D., & Szalay, A. S. 1993, Astrophysical Journal, 412, 64.
↑4	$4\pi r^2$ is the surface area of a sphere. When multiplied by the infinitesimal thickness $dr$ it becomes the volume of a very thin spherical shell.
↑5	A plane (of galaxies in this instance) intersected with a spherical shell creates a circular ring. The circumference of that ring is $2\pi r$ . When multiplied by the ring’s thickness $dr$ we get the area of the ring.
↑6	Though it might seem counterintuitive, the distribution of galaxies is considered to be a random process. That is, there could be an infinite number of different Universes that each have the same 2PCF. This is analogous to many people rolling a die a large number of times. Each person will roll numbers 1 through 6 in a different order even though the probability of rolling each number is identical for all of them. In fact, simulating the positions of galaxies is sometimes referred to as rolling the dice.
↑7	I have taken the radius of the sphere to increase by a factor of 2, but note that the argument works for any factor $f$ , e.g. changing the radius of the sphere from $R$ to $fR$ scales the area of the intersecting plane by $f^{D=2}$ .
↑8	The fractal dimension is also a measure of the complexity of a fractal’s boundary. There are formal defintions of $F_D$ , but those are omitted here.
↑9	The top-hat window function equals 1 when $x$ is within a distance $R$ of $x_0$ and equals zero otherwise. It exists to limit the integration to the interior of the sphere.
↑10	For conventional three-dimensional objects $N=3$ . When integrating over surfaces we use $N=2$ .
↑11	The approximation of the 2PCF as a power law works well for intermediate length scales. At small separations (e.g. the size of galaxies) the growth of structure is governed by factors far more complicated than simple gravity like supernovae, shockwaves, tidal forces, accretion disks, etc. At large separations parcels of matter are so distant that they have yet to have time to affect each other.

1 Comment

Robert L. Oldershaw Reply

August 2, 2016 at 9:15 pm

A new website relating to fractals and the study of fractal modeling of nature can be found at: http://fractalogy.org/ .

The site is still under development (by Bernard Geiger) but is fully functional and looking for interested people to join the discussions, especially professional physics/cosmology scientists.

Here is a link to a nice video intro to fractals and fractalogy.org: http://www.fractalogy.org/forum/viewtopic.php?f=8&t=58

I hope you will find the site well worth a look.

About Author

Mike Specian

Related Posts

1 Comment

Leave a Reply